The different sizes of infinity

2023-05-18 19:48:20

Since humanity has gazed at the stars, the concept of infinity has entered our minds with force. What is beyond our imagination, what is unattainable and incomprehensible… All of this, and much more, is actually infinity.

But infinity in mathematics goes a long way. the german mathematician Georg Cantor has been the person who has studied this concept most intensely and the person who, as I tell in my book history of infinityhas managed to tame the beast.

Cantor had, among others, arguments that allowed him to ensure that there are infinities of different sizes. How is it possible?

Gerog Cantor around 1870 (left), just at the beginning of his career as a mathematician, and around 1915 (right) at the end of his life.
Wikipedia

The infinity of the countable

To compare sizes of sets, the probably easiest technique is match objects from each other. If each element of a set is paired with an element of the other (and none are left over), we can ensure that both sets have the same number of elements, that is, they have the same size. In mathematics we say that we have established a bijection.

This matching technique works very well with finite sets. Counting things is nothing more than establishing a bijection between the first natural numbers and what we want to count.

But bijections are also a good way to compare sizes of infinite sets. And if we talk about infinity, the set that first comes to mind is that of all los natural numbers. Any set that can be put into bijection with the naturals is said to be numerable.

But our intuition can play tricks on us. Let’s see some of the examples that Cantor himself came across.

Los rational numbers are the fractions formed by whole numbers (avoiding zero in the denominator). In this way, all natural numbers are, in particular, rational. But… how many rational are there?

One of the first results about infinity that Cantor proved, while still a student and just for fun, was that, in reality, there are as many natural numbers as there are rational numbers. And this despite the fact that one is a part of the other.

Checking this is equivalent to giving an enumeration of the rationals, that is, establishing which is the first, the second, etc… To do this, the first thing is to realize that it is enough to list the positive rationals (of course, because we can always do: zero, positive, negative, positive, negative,…).

The argument attributed to Cantor is simple, but effective. We put all the rationals with denominator 1 in a row, in the second row those with denominator 2, in the third row those with denominator 3 and so on. In this way we will have all the rationals in a kind of endless table, although there will be many that appear repeated (it doesn’t matter, they will be removed later). Now, a simple zigzag line going through all the numbers in the table will act as an enumeration.

Zigzag enumeration of positive rational numbers.
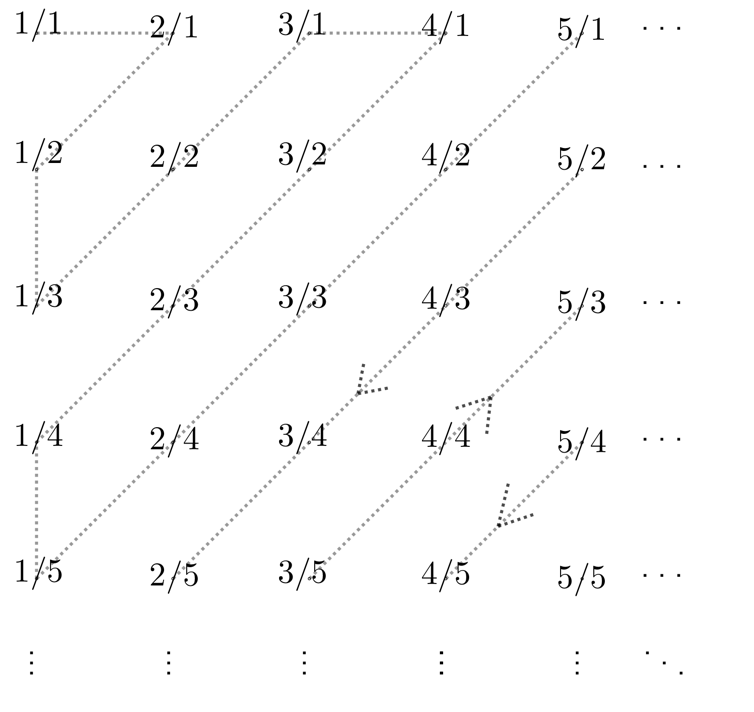
own elaboration

There are, then, as many natural numbers as there are rational numbers.

The infinity of the continuum

But if we draw all the rational numbers on the line, it turns out that they practically fill it. Actually, as close as we want to any (real) number there is a rational. This property is known as density from rationals to reals.

So Cantor, well acquainted with this fact, wondered if it would be possible to enumerate all the real numbers. Since it seems that what the rationals lack to fill the line is practically nothing, it is a most natural question (pun intended).

We are going to keep not all the real numbers, but those that are between 0 and 1. These numbers have the peculiarity that all They can be written in decimal form starting with 0 (remember that 1=0.999…).

Cantor proposes the following argument. Suppose we can enumerate all the real numbers between 0 and 1 and put them in a list. For example:

An enumeration of reals between 0 and 1.
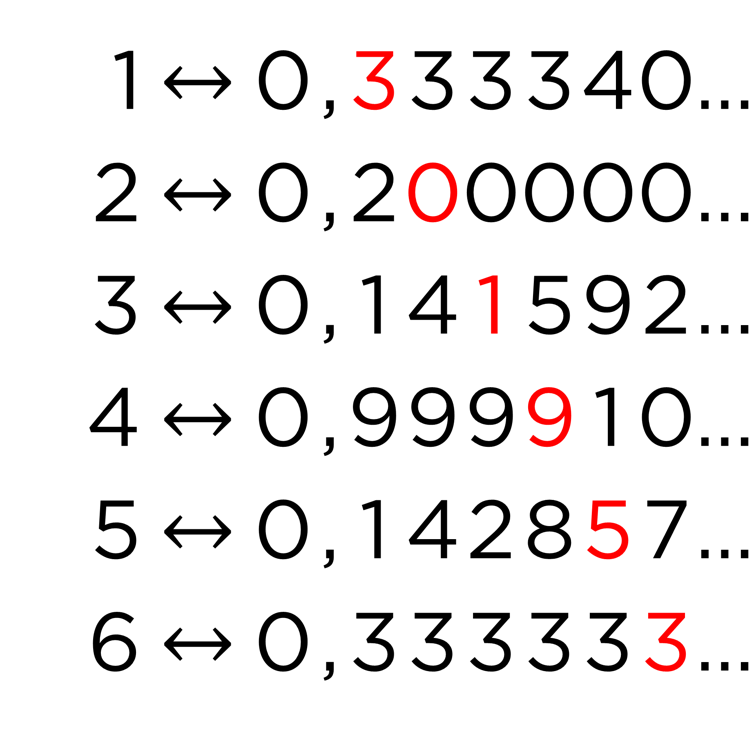
own elaboration

Well, based on that list, Cantor builds a new number.

Let’s look at the first decimal of the first number (the one in red), 3 in this case. For his number, Cantor takes as the first decimal any digit that no let 3 be, for example, 4.

We repeat the argument with the second decimal of the second number, 0; Cantor takes, for example, 1. Since the third decimal of the third number is 1, we take 2 for our number, for example. And so on, we continue with all the numbers on the list. In this way, we build the number 0.412064…

But what’s so special about that number? so what no it’s on the list. Indeed, since the first decimal is different from the first number in the list, it cannot be the first; since the second decimal is different from the second in the list, it cannot be the second either; nor the third, since it differs from the third decimal; Not the fourth, not the fifth…

In short, what Cantor demonstrates is that whatever the way of enumerating the numbers between 0 and 1, we are always going to miss at least one. In other words, it turns out impossible establish a bijection between the reals (between 0 and 1) and the naturals.

And since there are more real than natural numbers, Cantor concludes that the infinity of the continuum, that of the real numbers, is greater than the infinity of the natural numbers.

We have infinities of different sizes.

A whole succession of infinities each one bigger than the previous one

This Cantor argument was not the first he published. The original was much more complicated and technical and it brought many setbacks (you can also read this story in history of infinity).

To make up for it, the diagonal argument (as the one we have seen is known) actually offers a way of generating an infinity larger than any given. It is what is known as Cantor’s Theorem.

Cantor not only establishes the existence of an infinity greater than another. He shows that there is a whole succession of infinities, each one greater than the last.